Imagine a world where numbers can be more than just simple quantities. A world where numbers have their own hidden depths, mysteries, and secrets. Welcome to the fascinating realm of complex numbers! These are not mere mathematical curiosities; they are essential tools in various fields, from electrical engineering to quantum mechanics. In this study guide and intervention, we’ll explore the fundamentals of complex numbers, uncovering their intricate nature and unlocking the power they hold.
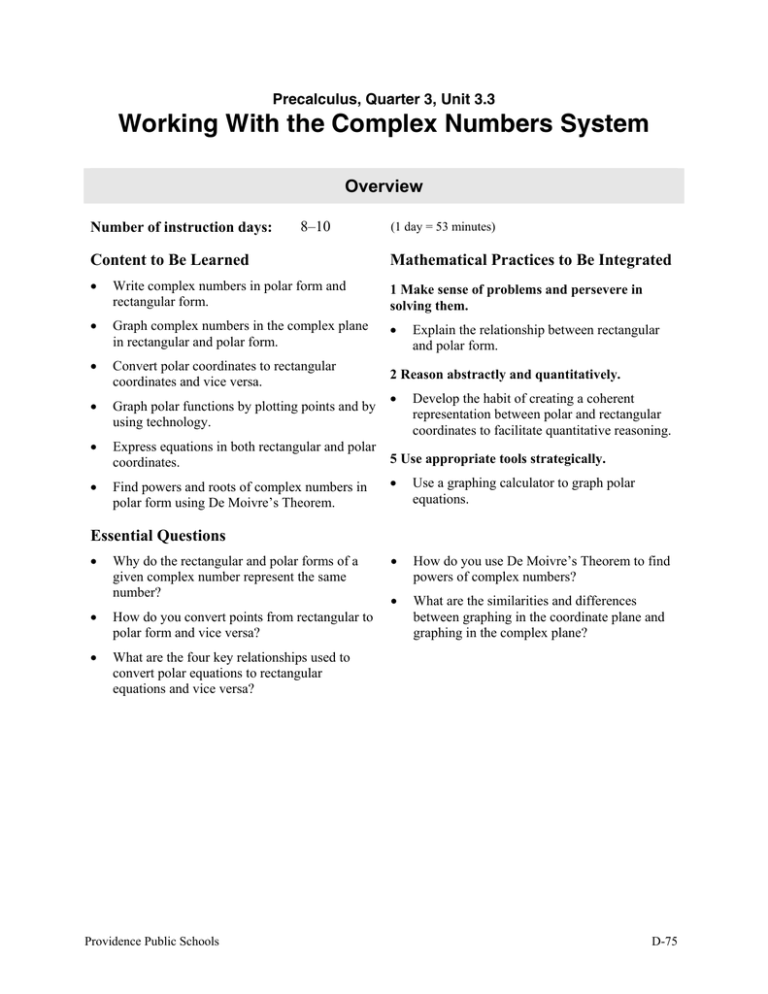
Image: studylib.net
But first, let’s address the elephant in the room: why do we need complex numbers? After all, we already have real numbers – those familiar digits we use for counting, measuring, and calculating. Well, just as the real number line represents all possible magnitudes, complex numbers expand the mathematical landscape, incorporating the concept of direction. This opens up a whole new dimension of possibilities, allowing us to solve equations that were previously unsolvable, analyze intricate systems, and understand complex phenomena in a deeper way.
What Are Complex Numbers?
At their core, complex numbers are defined as numbers of the form a + bi, where ‘a‘ and ‘b‘ are real numbers, and ‘i‘ is the imaginary unit. This imaginary unit, ‘i‘, is defined as the square root of -1. If you’ve ever wondered how to take the square root of a negative number, ‘i‘ is the answer. It introduces an entirely new dimension to our number system, allowing us to work with negative square roots and explore the world beyond the real number line.
The Complex Plane
To visualize complex numbers effectively, we use the complex plane. This is similar to the familiar Cartesian coordinate plane but with a twist. The horizontal axis represents the real part (a) of the complex number, while the vertical axis represents the imaginary part (b). Each point in this plane corresponds to a unique complex number, providing a graphical representation of the entire complex number system.
The Magic of ‘i’
The imaginary unit, ‘i‘, is the heart and soul of complex numbers. Its property, i² = -1, unlocks a whole range of possibilities within this number system. With this fundamental definition, we can perform arithmetic operations with complex numbers, including addition, subtraction, multiplication, and division.
Image: www.chegg.com
Working with Complex Numbers
Let’s dive into the fascinating world of manipulating complex numbers. We’ll explore the various operations and their applications:
1. Addition and Subtraction
Adding or subtracting complex numbers is very straightforward. We simply add or subtract the corresponding real and imaginary parts:
(a + bi) + (c + di) = (a + c) + (b + d)i
2. Multiplication
Multiplying complex numbers involves using the distributive property and remembering that i² = -1.
(a + bi) × (c + di) = ac + adi + bci + bdi²
Simplifying, we get:
(a + bi) × (c + di) = (ac – bd) + (ad + bc)i
3. Division
Dividing complex numbers requires a bit more finesse. We use a technique called “rationalization” to eliminate the imaginary unit from the denominator. This involves multiplying both the numerator and denominator by the complex conjugate of the denominator. The complex conjugate of a complex number ‘a + bi‘ is ‘a – bi‘.
Applications of Complex Numbers
While complex numbers might seem abstract, they are deeply rooted in the real world. Here are a few examples:
1. Electrical Engineering
Complex numbers are crucial for understanding and analyzing alternating current (AC) circuits. They simplify calculations involving impedance, a measure of the opposition to the flow of alternating current. Using complex numbers, engineers can analyze circuit behavior, determine power consumption, and design efficient electrical systems.
2. Quantum Mechanics
The bizarre world of quantum mechanics relies heavily on complex numbers. These numbers are used to represent the wave functions of particles, describing their probability distributions and behaviors. Quantum calculations, such as those involving wave interference and particle spin, are heavily reliant on complex number theory.
3. Signal Processing
Signal processing, a fundamental aspect of modern communication technology, relies extensively on the power of complex numbers. They help analyze, process, and manipulate signals, making it possible to filter out noise, compress data, and effectively transmit information.
4-4 Study Guide And Intervention Complex Numbers
Conclusion
The world of complex numbers is a fascinating and intricately woven tapestry, expanding the boundaries of mathematics and opening a gateway to deeper understanding in various fields. By understanding their properties, how to work with them, and their widespread applications, we gain a powerful tool for solving complex problems, analyzing intricate systems, and uncovering hidden secrets within the world around us.
This Study Guide and Intervention has provided a foundation for your journey into the realm of complex numbers. Now, it’s your turn to explore further. Dive into the depths of complex number theory, delve into its applications, and discover the hidden beauty and power within this remarkable mathematical construct.





