Remember that intense feeling of dread before a big test? The butterflies in your stomach, the shaky hands, and the overwhelming sense of “I’m not ready!” Well, that’s exactly how I felt before my Unit 8 test on quadratic equations. Quadratics seemed like a whole new beast, with their strange graphs, confusing formulas, and endless word problems. But then, I discovered the power of a well-structured study guide, and everything changed.
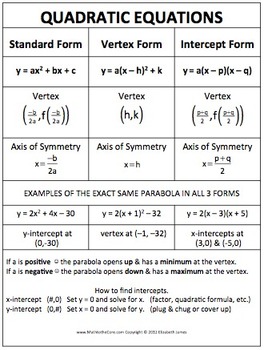
Image: www.myxxgirl.com
This guide isn’t just about memorizing formulas – it’s about understanding the “why” behind the “what,” and gaining the confidence to tackle any quadratic equation that comes your way. We’ll cover the basics, explore different solution methods, and unveil those hidden patterns that make quadratics more predictable than you might think. So, buckle up, grab your notebook, and let’s conquer this unit together!
Understanding the Basics: What are Quadratic Equations?
At its core, a quadratic equation is a polynomial equation where the highest power of the variable is 2. This means you’ll be dealing with terms like “x²” and “ax²” (where “a” is a constant). These equations are often encountered in real-world applications, from calculating the trajectory of a ball to modeling the growth of a population.
The standard form of a quadratic equation is ax² + bx + c = 0, where a, b, and c are coefficients, and ‘a’ can’t be zero (otherwise, it wouldn’t be quadratic!). This form is crucial for applying different solving techniques.
Solving Quadratic Equations – Unlocking the Secrets
The Power of Factoring
Factoring is like breaking a puzzle into its pieces. If you can factor a quadratic equation, you can quickly find the values of x that make the equation true (these values are called roots or solutions). The key is to find two binomials that multiply to equal the original quadratic expression. For example, x² + 5x + 6 can be factored into (x + 2)(x + 3). The roots of this equation are -2 and -3, as they make the equation true.

Image: studyfinder.org
The Reliable Quadratic Formula
Sometimes, factoring isn’t the easiest route. That’s where the quadratic formula comes in handy. It’s a universal solution, working for any quadratic equation even if it can’t be factored. The formula is: x = (-b ± √(b² – 4ac)) / 2a. It might look intimidating, but it’s simply a recipe for finding the roots. Just plug in the coefficients (a, b, and c) and simplify.
Completing the Square
Completing the square is a method for rewriting the quadratic equation in a way that allows you to directly solve for x. It involves manipulating the equation to create a perfect square trinomial. This method can be a bit more involved, but it’s a valuable tool for understanding the structure of quadratic equations.
The Nature of Roots: Discrimant to the Rescue
The discriminant (b² – 4ac) is like a detective, revealing crucial information about the roots of a quadratic equation. If the discriminant is positive, the equation has two distinct real roots. If it’s zero, there’s one repeated real root. And if it’s negative, there are two complex roots (involving imaginary numbers). Knowing the discriminant can help you predict the types of solutions you’ll find, saving you time and frustration.
The World of Quadratics: A Look at Applications
Quadratic equations are not just mathematical curiosities – they have practical applications in various fields.
Physics: Projecting Motion
In physics, quadratic equations are used to model projectile motion. Think about throwing a ball – its trajectory can be described by a quadratic equation, considering factors like initial velocity, angle, and gravity. The equation helps predict when and where the ball will hit the ground!
Finance: Calculating Interest
Financial models often involve quadratic equations when dealing with compound interest. Understanding how these equations work is crucial for making smart investment decisions.
Engineering: Designing Shapes
Quadratic equations are vital in engineering to design curved shapes, for example, bridges or parabolic antennas. By using quadratic functions, engineers can ensure the structural integrity and functionality of these designs.
Quadratic Equations: Latest Trends
Quadratic equations are a timeless topic in mathematics, but research continues to explore their applications in various fields. For example:
- Advancements in Computer Science: Quadratic equations are used in algorithms and optimization problems. Areas like artificial intelligence and machine learning rely on efficient quadratic equation solving techniques.
- New Areas of Application: Researchers are exploring the use of quadratic equations in fields like bioinformatics and cryptography, uncovering new ways to model complex systems and secure data.
- Innovative Teaching Methods: Educational technology is incorporating interactive simulations and online tools to make learning quadratic equations more engaging and accessible.
Tips and Expert Advice to Dominate Your Test
Now that you have a good grasp of the concepts, here’s some expert advice to help you ace your Unit 8 test:
- Practice, Practice, Practice: The key to mastering quadratic equations is repetition. Work through diverse examples, covering all the solving methods and real-world applications.
- Visualize and Understand: Don’t just memorize formulas. Try to visualize the graphs of quadratic equations and understand how the coefficients affect their shape and position.
- Seek Help: Don’t hesitate to ask your teacher or classmates for help if you’re stuck. There’s no shame in seeking extra support – it can actually make a huge difference in your understanding.
- Stay Organized: Keeping your notes, practice problems, and formulas organized will help you find information quickly and efficiently when studying.
Remember, studying isn’t just about cramming information – it’s about building a strong foundation. Understanding the concepts behind quadratic equations will make applying them in different scenarios much easier.
FAQs About Quadratic Equations
Q: What’s the Difference Between a Quadratic Equation and a Quadratic Function?
A: A quadratic equation is an expression that’s set equal to zero, while a quadratic function is a general expression that defines a relationship between inputs and outputs. The equation focuses on solving for roots, while the function describes a curve on a graph.
Q: Can a Quadratic Equation Have More Than Two Roots?
A: No, a quadratic equation can have at most two roots. This is because the highest power of the variable is 2, and a polynomial equation’s degree determines the maximum number of roots.
Q: How Can I Graph a Quadratic Equation?
A: You can graph a quadratic equation by plotting points or using the vertex form of the equation. The vertex form helps locate the vertex of the parabola, which is the maximum or minimum point of the graph.
Unit 8 Test Study Guide Quadratic Equations
Conclusion
Quadratic equations may seem daunting at first, but with the right approach, they can become your allies. Understanding the definitions, solving methods, and real-world applications will empower you to tackle any problem with confidence. So, buckle up, practice diligently, and remember, success is within your reach!
Are you ready to conquer your Unit 8 test on quadratic equations? I know you can do it!





